Constructive Mathematics
Constructive mathematics is distinguished from its traditional
counterpart, classical mathematics, by the strict interpretation of
the phrase ‘there exists’ as ‘we can construct’.
In order to work constructively, we need to re-interpret not only the
existential quantifier but all the logical connectives and
quantifiers as instructions on how to construct a proof of the
statement involving these logical expressions.
Although certain individuals -- most notably Kronecker -- had expressed
disapproval of the ‘idealistic’, nonconstructive methods
used by some of their nineteenth century contemporaries, it is in the
polemical writings of L.E.J. Brouwer (1881-1966), beginning with his
Amsterdam doctoral thesis (Brouwer 1907) and continuing over the
next forty-seven years, that the foundations of a precise, systematic
approach to constructive mathematics were laid. In Brouwer's
philosophy, known as intuitionism, mathematics is
a free creation of the human mind, and an object exists if and only
if it can be (mentally) constructed.
Before mathematicians assert something they are supposed to have
proved it true. What, then, do mathematicians mean when they assert a
disjunction
P 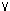 Q, where P and Q are syntactically
correct statements in some (formal or informal) language that a
mathematician can use? A natural -- although, as we shall see, not the
unique -- interpretation of this disjunction is that not only does (at
least) one of the statements P, Q hold, but also we
can decide which one holds. Thus just as mathematicians will assert
that P only when they have decided that P by
proving it, they may assert
P
Q, where P and Q are syntactically
correct statements in some (formal or informal) language that a
mathematician can use? A natural -- although, as we shall see, not the
unique -- interpretation of this disjunction is that not only does (at
least) one of the statements P, Q hold, but also we
can decide which one holds. Thus just as mathematicians will assert
that P only when they have decided that P by
proving it, they may assert
P 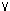 Q only when they either can decide -- that is, prove -- that
P or decide (prove) that Q.
Q only when they either can decide -- that is, prove -- that
P or decide (prove) that Q.
With this interpretation, however, mathematicians run into a serious
problem in the special case where Q is the negation,
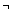 P,
of P. To decide that
P,
of P. To decide that
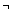 P
is to show that P implies a contradiction (such as
0=1). But it will often be that mathematicians have
neither decided that P nor decided that
P
is to show that P implies a contradiction (such as
0=1). But it will often be that mathematicians have
neither decided that P nor decided that
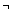 P.
To see this, we need only reflect on the following:
P.
To see this, we need only reflect on the following:
Goldbach Conjecture:
Every even integer > 2 can be written as a sum of two primes,
which remains neither proved nor disproved despite the best efforts
of many of the leading mathematicians since it was first raised in a
letter from Goldbach to Euler in 1742. We are forced to conclude
that, under the very natural interpretation of
P
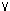 Q just canvassed, only an optimist can retain a belief in
the law of excluded middle,
P
Q just canvassed, only an optimist can retain a belief in
the law of excluded middle,
P 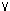
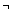 P.
P.
Traditional, or classical, mathematics gets round this by
widening the interpretation of disjunction: it interprets
P 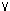 Q as
Q as
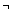 (
(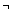 P
P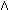
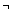 Q), or
in other words, “it is contradictory that both P
and Q be false”. In turn, this leads to the
idealistic interpretation of existence, in which
Q), or
in other words, “it is contradictory that both P
and Q be false”. In turn, this leads to the
idealistic interpretation of existence, in which
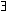 xP(x) means
xP(x) means
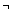
 x
x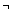 P(x)
(“it is contradictory that P(x) be false for
every x”). It is on these interpretations of
disjunction and existence that mathematicians have built the grand,
and apparently impregnable, edifice of classical mathematics which
serves a foundation for the physical, the social, and (increasingly)
the biological sciences. However, the wider interpretations come at a
cost: for example, when we pass from our initial, natural
interpretation of
P
P(x)
(“it is contradictory that P(x) be false for
every x”). It is on these interpretations of
disjunction and existence that mathematicians have built the grand,
and apparently impregnable, edifice of classical mathematics which
serves a foundation for the physical, the social, and (increasingly)
the biological sciences. However, the wider interpretations come at a
cost: for example, when we pass from our initial, natural
interpretation of
P 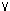 Q to the
unrestricted use of the idealistic one,
Q to the
unrestricted use of the idealistic one,
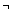 (
(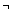 P
P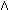
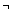 Q),
the resulting mathematics cannot generally be interpreted within
computational models such as recursive function theory.
Q),
the resulting mathematics cannot generally be interpreted within
computational models such as recursive function theory.
This point is illustrated by a well-worn example, the proposition:
There exists irrational numbers a, b such that
ab is rational.
A slick classical proof goes as follows. Either
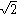
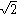 is rational, in which case we take a = b =
is rational, in which case we take a = b =
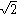 ; or else
; or else
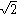
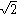 is irrational, in which case we take a =
is irrational, in which case we take a =
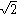
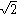 and
b =
and
b =
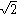 (See Dummett 1977, 10). But as it stands, this proof does not enable
us to pinpoint which of the two choices of the pair
(a,b) has the required property. In order to
determine the correct choice of (a,b), we would
need to decide whether
(See Dummett 1977, 10). But as it stands, this proof does not enable
us to pinpoint which of the two choices of the pair
(a,b) has the required property. In order to
determine the correct choice of (a,b), we would
need to decide whether
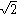
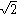 is rational or irrational, which is precisely to employ our initial
interpretation of disjunction with P the statement
“
is rational or irrational, which is precisely to employ our initial
interpretation of disjunction with P the statement
“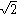
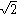 is rational”.
is rational”.
Here is another illustration of the difference between
interpretations. Consider the following simple statement about the
set 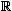 of real numbers:
of real numbers:
(*) 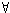 x
x
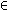
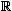 (x = 0
(x = 0 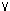 x
x 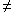 0),
0),
where, for reasons that we divulge shortly, x
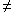 0 means that we can find a rational number r with 0 <
r < |x|. A natural computational interpretation
of (*) is that we have a procedure which, applied to any real number
x, either tells us that x=0 or else tells us that
x
0 means that we can find a rational number r with 0 <
r < |x|. A natural computational interpretation
of (*) is that we have a procedure which, applied to any real number
x, either tells us that x=0 or else tells us that
x
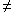 0. (For example, such a procedure might output 0 if x=0,
and output 1 if x
0. (For example, such a procedure might output 0 if x=0,
and output 1 if x
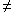 0.) However, because the computer can handle real numbers only by
means finite rational approximations, we have the problem of
underflow, in which a sufficiently small positive number can
be misread as 0 by the computer; so there cannot be a decision
procedure that justifies the statement (*). In other words, we cannot
expect (*) to hold under our natural computational interpretation of
the quantifier
0.) However, because the computer can handle real numbers only by
means finite rational approximations, we have the problem of
underflow, in which a sufficiently small positive number can
be misread as 0 by the computer; so there cannot be a decision
procedure that justifies the statement (*). In other words, we cannot
expect (*) to hold under our natural computational interpretation of
the quantifier
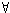 and the connective
and the connective
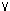 .
.
Let's examine this from another angle. Let
G(n) act as shorthand for the statement
“2n + 2 is a sum of two primes”, where n
ranges over the positive integers, and define an infinite binary
sequence a =
(a1,a2,…) as follows:
| an = |
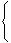 |
0
1 |
if G(n) holds for all k
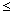 n n
if 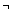 G(n) holds for some k G(n) holds for some k
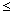 n n |
There is no question that a is a computationally
well-defined sequence, in the sense that we have an algorithm for
computing an for each n: check
the even numbers 4,6,8,…,2n+2 to determine whether each
of them is a sum of two primes; in that case, set
an=0; in the contrary case, set
an=1. Now consider the real number whose
nth binary digit is
an:
| x |
= |
(0 a1a2a2 a1a2a2   )2 )2 |
|
= |
2-1a1 + 2-2a2 +    |
|
= |
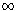
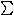
n=1 |
2-nan.
|
|
If (*) holds under our computational interpretation, then we can
decide between the following two alternatives:
- 2-1a1 +
2-2a2 +


 = 0, which implies that an = 0 for
every n;
= 0, which implies that an = 0 for
every n;
- we can find a positive integer N such that
2-1a1 +
2-2a2 +


 >
2-N.
>
2-N.
In the latter case, by testing
a1,…,aN, we can
find n
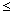 N such that an = 1. Thus the
computational interpretation of (*) enables us to decide whether
there exists n such that an =
1; in other words, it enables us to decide the status of the Goldbach
Conjecture.
N such that an = 1. Thus the
computational interpretation of (*) enables us to decide whether
there exists n such that an =
1; in other words, it enables us to decide the status of the Goldbach
Conjecture.
The use of the Goldbach Conjecture here is purely dramatic. To avoid
it, we define a function f on the set of binary sequences as
follows:
| f(a) = |
 |
0
1 |
if an = 0 for all n
if an = 1 for some n. |
The argument of the preceding paragraph can then be modified to show
that, under our computational interpretation, (*) provides us with a
procedure for calculating f(a) for any
computationally well-defined binary sequence
f(a). Now, the computability of the function
f can be expressed informally by the following:
Limited Principle of Omniscience (LPO):
For each binary sequence
(a1,a2,…) either
an = 0 for all n or else there
exists n such that an =
1,
which is generally regarded as an essentially nonconstructive
principle, for several reasons. First, its recursive
interpretation,
There is a recursive algorithm which, applied to any recursively
defined binary sequence
(a1,a2,…), outputs 0 if
an = 0 for all n, and outputs 1
if an = 1 for some n,
is provably false within recursive function theory, even with
classical logic (see Bridges & Richman 1987, Chapter 3); so if we
want to allow a recursive interpretation of all our mathematics, then
we cannot use LPO. Secondly, there is a model theory (involving the
use of Kripke models) in which it can be shown that LPO is not
derivable in Peano arithmetic using the computational interpretation
of the connectives and quantifiers that we state in more detail in
the next section (Bridges & Richman 1987, Chapter 7).
It should, by now, be clear that a full-blooded computational
development of mathematics disallows the idealistic interpretations
of disjunction and existence upon which most classical mathematics
depends. In fact, in order to work constructively, we need to return
from the classical interpretations back to the natural, constructive
ones, as follows.
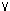 (or): (or): |
to prove P
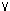 Q we must have either a proof of P or a proof of
Q.
Q we must have either a proof of P or a proof of
Q. |
 (and): (and): |
to prove P
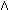 Q we must have a proof of P and a proof of
Q.
Q we must have a proof of P and a proof of
Q. |
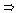 (implies): (implies): |
a proof of P
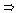 Q is an algorithm that converts a proof of P into
a proof of Q.
Q is an algorithm that converts a proof of P into
a proof of Q. |
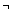 (not): (not): |
to prove
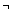 P we must show that P implies 0
= 1. P we must show that P implies 0
= 1. |
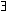 (there exists): (there exists): |
to prove
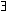 xP(x) we must construct an
object x and prove that P(x)
holds. xP(x) we must construct an
object x and prove that P(x)
holds. |
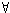 (for each/all): (for each/all): |
a proof of
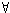 xP(x)
is an algorithm that, applied to any object x,
proves that P(x) holds. xP(x)
is an algorithm that, applied to any object x,
proves that P(x) holds. |
These computational interpretations can be made more precise using
Kleene's notion of realizability; see
(Dummett 1977, 318-335; Beeson 1985, Chapter
VII).
Why would we want to do this? First, there is the desire to retain,
as far as possible, computational interpretations of our
mathematics. Ideally, we are trying to develop mathematics in such a
way that if a theorem asserts the existence of an object x
with a property P, then the proof of the theorem embodies
algorithms for constructing x and for demonstrating, by
whatever calculations are necessary, that x has the property
P. Here are some examples of theorems, each followed by an
informal description of the requirements for its constructive
proof.
| (A) |
For each real number x, either x = 0 or
x
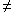 0. 0. |
|
Proof requirement:
An algorithm which, applied to a given real number x,
would decide whether x = 0 or x
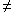 0. Note that, in order to make this decision,
the algorithm might use not only the data describing x but
also the data showing that x is actually a real
number. 0. Note that, in order to make this decision,
the algorithm might use not only the data describing x but
also the data showing that x is actually a real
number. |
| (B) |
Each nonempty subset S of R that is
bounded above has a least upper bound. |
|
Proof requirement: An algorithm which, applied to a set
S of real numbers, a member s of S, and an
upper bound for S,
- computes an object b and shows that b is a real
number;
- shows that x
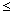 b for each x
b for each x
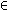 S; and S; and
- given a real number
b
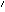 < b, computes an element x of S such
that x >
b
< b, computes an element x of S such
that x >
b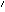 . .
|
| (C) |
If f is a continuous real-valued mapping on the closed
interval [0,1] such that f(0)f(1) < 0, then
there exists x such that 0 < x < 1 and
f(x) = 0. |
|
Proof requirement: An algorithm which, applied to the
function f, a modulus of continuity for f, and the
values f(0) and f(1),
- computes an object x and shows that x is a
real number between 0 and 1, and
- shows that f(x) = 0.
|
| (D) |
If f is a continuous real-valued mapping on the closed
interval [0,1] such that f(0)f(1) < 0, then
for each
 > 0 there exists x such
that 0 < x < 1 and
|f(x)| <
> 0 there exists x such
that 0 < x < 1 and
|f(x)| <
 . . |
|
Proof requirement: An algorithm which, applied to the function
f, a modulus of continuity for f, the values
f(0) and f(1), and a positive number
 , ,
- computes an object x and shows that x is a
real number between 0 and 1, and
- shows that |f(x)| <
 . .
|
We already have reasons for doubting that (A) has a constructive
proof. If the proof requirements for (B) can be fulfilled, then,
given a binary sequence
(a1,a2,…), we can apply
our proof of (B) to the set
{a1,a2,…} in order to
determine its supremum
 . Computing
. Computing
 with an error < 1/2, we then determine whether
with an error < 1/2, we then determine whether
 = 0 or
= 0 or
 = 1; in the first case, an = 0 for all
n, whereas in the second, we can easily find N such
that aN = 1. Thus (B) implies LPO and is
therefore essentially nonconstructive. However, in Bishop's
constructive theory of the real numbers, based on Cauchy sequences
with a preassigned convergence rate, we can prove the following:
= 1; in the first case, an = 0 for all
n, whereas in the second, we can easily find N such
that aN = 1. Thus (B) implies LPO and is
therefore essentially nonconstructive. However, in Bishop's
constructive theory of the real numbers, based on Cauchy sequences
with a preassigned convergence rate, we can prove the following:
Constructive Least-Upper-Bound Principle:
Let S be a nonempty subset of R that is bounded
above. Then S has a least upper bound if and only if it is
located, in the sense that for all real
numbers
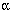 ,
,
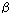 with
with
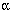 <
< 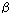 ,
either
,
either
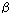 is an upper bound for S or else there exists x in>
S with x >
is an upper bound for S or else there exists x in>
S with x > 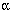 (Bishop &
Bridges 1985, p. 37, Proposition (4.3))
(Bishop &
Bridges 1985, p. 37, Proposition (4.3))
Each of statements (C) and (D), which are classically equivalent, is
a version of the Intermediate Value Theorem. In these statements, a
modulus of continuity for f is a set
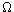 of ordered pairs
(
of ordered pairs
( ,
,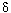 )
of positive real numbers with the following two properties:
)
of positive real numbers with the following two properties:
- for each
 > 0 there exists
> 0 there exists
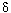 > 0 such that
(
> 0 such that
( ,
,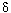 )
)
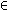
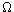 ;
;
- for each
(
 ,
,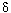 )
)
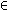
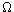 ,
and for all x,y
,
and for all x,y
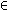 [0,1] such that
|x - y| <
[0,1] such that
|x - y| <
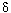 , we have
|f(x) - f(y)| <
, we have
|f(x) - f(y)| <
 .
.
Statement (C) is essentially nonconstructive, since it entails the
following nonconstructive principle:
Lesser Limited Principle of Omniscience (LLPO):
For each binary sequence
(a1,a2,…) with at most
one term equal to 1, either an = 0 for
all even n or else an = 0
for all odd n.
Statement (D), a weak form of (C), can be proved constructively,
using an interval-halving argument of a standard type. The following
stronger constructive intermediate value theorem, which suffices for
most practical purposes, is proved using an approximate
interval-halving argument:
Let f be a continuous real-valued mapping on the closed
interval [0,1] such that f(0) and f(1) have
opposite signs. Suppose also that f is locally
nonzero, in the sense that for each x
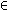 [0,1] and each r > 0, there exists y such
that
|x - y| < r and f(y)
[0,1] and each r > 0, there exists y such
that
|x - y| < r and f(y)
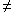 0. Then there exists x such that 0 < x < 1
and f(x)=0.
0. Then there exists x such that 0 < x < 1
and f(x)=0.
The situation of the intermediate value theorem is typical of many
in constructive analysis, where we find one classical theorem with
several constructive versions, some or all of which may be equivalent
under classical logic. (See also, for example, Bridges et al.
1982.)
There is one omniscience principle whose constructive status is less
clear than that of LPO and LLPO, namely, the following:
Markov's Principle (MP):
For each binary sequence (an), if it is
contradictory that all the terms an
equal 0, then there exists a term equal to 1.
This principle is equivalent to a number of simple classical
propositions, including the following:
- For each real number x, if it is contradictory that
x equal 0, then x
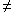 0 (in the sense we mentioned earlier).
0 (in the sense we mentioned earlier).
- For each real number x, if it is contradictory that
x equal 0, then there exists y
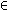
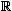 such that xy = 1.
such that xy = 1.
- For each one-one continuous mapping f : [0,1]
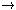
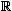 ,
if x
,
if x
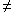 y, then f(x)
y, then f(x)
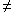 f(y).
f(y).
Markov's Principle represents an unbounded search: if you have a
proof that all terms an being 0 leads to
a contradiction, then, by testing the terms
a1,a2,a3,…
in turn, you are ‘guaranteed’ to come across a term equal
to 1; but this guarantee does not extend to an assurance that you
will find the desired term before the end of the universe. Most
practitioners of constructive mathematics view Markov's Principle
with at least suspicion, if not downright disbelief. Such views are
reinforced by the observation that there is a Kripke Model showing
that MP is not derivable in Peano arithmetic under our computational
interpretation of logic. (See Bridges & Richman 1987, 137-138.)
The desire to retain the possibility of a computational
interpretation is one motivation for using the constructive
reinterpretations of the logical connective and quantifiers that we
gave above; but it is not exactly the motivation of the pioneers of
constructivism in mathematics.
In the late nineteenth century, certain individuals -- most notably
Kronecker and Poincaré -- had expressed doubts, or even
disapproval, of the idealistic, nonconstructive methods used by some
of their contemporaries; but it is in the polemical writings of
L.E.J. Brouwer (1881-1966), beginning with his Amsterdam doctoral
thesis in 1907 and continuing over the next forty-seven years, that
the foundations of a precise, systematic approach to constructive
mathematics were laid. In Brouwer's philosophy, known as
intuitionism, mathematics is a free creation of the human
mind, and an object exists if and only if it can be (mentally)
constructed. If one takes that philosophical stance, then one is
inexorably drawn to the foregoing constructive interpretation of the
logical connectives and quantifiers: for how could a proof of the
impossibility of the non-existence of a certain object x --
an idealistic proof of the existence of x -- describe a
mental construction of x?
Brouwer was not the clearest expositor of his ideas, as is shown by the
following quotation:
Mathematics arises when the subject of two-ness, which results from
the passage of time, is abstracted from all special occurrences. The
remaining empty form [the relation of n to n+1] of
the common content of all these two-nesses becomes the original
intuition of mathematics and repeated unlimitedly creates new
mathematical subjects. (quoted in Kline 1972, pp. 1199-2000)
A modern precis of Brouwer's view was given by Errett Bishop
(Bishop 1967, p. 2):
The primary concern of mathematics is number, and this means the
positive integers. We feel about number the way Kant felt about
space. The positive integers and their arithmetic are presupposed by
the very nature of our intelligence and, we are tempted to believe,
by the very nature of intelligence in general. The development of the
positive integers from the primitive concept of the unit, the concept
of adjoining a unit, and the process of mathematical induction
carries complete conviction. In the words of Kronecker, the positive
integers were created by God.
However obscure Brouwer's writings could be, one thing was always
clear: for him, mathematics took precedence over logic. One might
say, as Hermann Weyl does in the following passage, that Brouwer saw
classical mathematics as flawed precisely in its use of classical
logic without reference to the underlying mathematics:
According to [Brouwer's] view and reading of history, classical
logic was abstracted from the mathematics of finite sets and their
subsets. ... Forgetful of this limited origin, one afterwards
mistook that logic for something above and prior to all mathematics,
and finally applied it, without justification, to the mathematics of
infinite sets. This is the Fall and original sin of set theory, for
which it is justly punished by the antinomies. It is not that such
contradictions showed up that is surprising, but that they showed up
at such a late stage of the game. (quoted in Kline 1972, p. 2001)
In particular, this misuse of logic led to nonconstructive existence
proofs which, in Hermann Weyl's words, “inform the world
that a treasure exists without disclosing its location”.
In order to describe the logic used by the intuitionist
mathematician, it was necessary first to analyse the mathematical
processes of the mind, from which analysis the logic could be
extracted. In 1930, Brouwer's most famous pupil, Arend Heyting,
published a set of formal axioms which so clearly characterise the
logic used by the intuitionist that they have become universally
known as the axioms for intuitionistic logic (Heyting 1930). These
axioms captured the informal computational interpretations of the
connectives and quantifiers that we gave earlier. Over the years,
Brouwer added principles, suggested by his introspective analysis of
the nature of the continuum, which made intuitionistic mathematics
diverge dramatically from its classical counterpart. The reader is
referred to the entry on
intuitionistic logic
for more information about such matters.
Unfortunately -- and perhaps inevitably, in the face of opposition
from mathematicians of such stature as Hilbert -- Brouwer's
intuitionist school of mathematics and philosophy became more and
more involved in what, at least to classical mathematicians, appeared
to be quasi-mystical speculation about the nature of constructive
thought, to the detriment of the practice of constructive mathematics
itself. On the other hand, in the late 1940s the Russian
mathematician A.A. Markov (Markov 1954) began the development of a form of
recursive constructive mathematics (RUSS), which was, essentially,
recursive function theory with intuitionistic logic (see Kushner
1985). In this variety the objects are defined by means of
Gödel-numberings, and the procedures are all recursive; the main
distinction between RUSS and the classical recursive analysis
developed after, in 1936, the work of Turing, Church, and others
clarified the nature of computable processes, is that the logic used
in RUSS is intuitionistic. Thus RUSS may be described as ‘recursive
mathematics with intuitionistic logic’.
One obstacle faced by the mathematician attempting to come to grips
with RUSS is that, expressed in the language of recursion theory, it
is not easily readable; indeed, on opening a page of Kushner's
excellent lectures, one might be forgiven for wondering whether this
is analysis or logic. Fortunately, one can get to the heart of RUSS
by an axiomatic approach, as shown in Richman 1983.
Progress in all varieties of constructive mathematics was relatively
slow throughout the next decade and a half. What was needed to raise
the profile of constructivism in mathematics was a top-ranking
classical mathematician to show that a thoroughgoing constructive
development of mathematics was possible without a commitment to
Brouwer's non-classical principles or to the machinery of recursive
function theory. This need was fulfilled in 1967, with the appearance
of Errett Bishop's monograph Foundations of Constructive
Analysis (Bishop 1967), the product of an astonishing couple of
years in which, working in the informal but rigorous style used by
normal analysts, Bishop provided a constructive development of a
large part of twentieth-century analysis, including the
Stone-Weierstrass Theorem, the Hahn-Banach and separation theorems,
the spectral theorem for self-adjoint operators on a Hilbert space,
the Lebesgue convergence theorems for abstract integrals, Haar
measure and the abstract Fourier transform, ergodic theorems, and the
elements of Banach algebra theory. (See also Bishop & Bridges
1985.) Thus, at a stroke, he gave the lie to the commonly-held view
expressed so forcefully by Hilbert:
Taking the principle of excluded middle from the mathematician would
be the same, say, as proscribing the telescope to the astronomer or
to the boxer the use of his fists. (Hilbert 1928)
Not only did Bishop's mathematics (BISH) have the advantage of
readability -- if you open Bishop's book at any page, what you see is
clearly recognisable as analysis, even if, from time to time, his
moves in the course of a proof may appear strange to one schooled in
the use of the law of excluded middle -- but, unlike intuitionistic
or recursive mathematics, it admits many different
interpretations. Intuitionistic mathematics, Markov's recursive
constructive mathematics, and even classical mathematics all provide
models of BISH. In fact, the results and proofs in BISH can be
interpreted, with at most minor amendments, in any reasonable model
of computable mathematics, such as, for example, Weihrauch's Type Two
Effectivity Theory (Weihrauch 1996, 2000).
How is this multiple interpretability achieved? At least in part by
Bishop's refusal to pin down his primitive notion of
‘algorithm’ or, in his words, ‘finite
routine’. This refusal has led to the criticism that his
approach lacks the precision that a logician would normally expect of
a foundational system. However, this criticism can be overcome by
looking more closely at what practitioners of BISH actually do, as
distinct from what Bishop may have thought he was doing, when they
prove theorems: in practice, they are doing mathematics with
intuitionistic logic. Experience shows that the restriction to
intuitionistic logic always forces mathematicians to work in a manner
that, at least informally, can be described as algorithmic; so
algorithmic mathematics appears to be equivalent to mathematics
that uses only intuitionistic logic. If that is the case, then
we can practice constructive mathematics using intuitionistic logic
on any reasonably defined mathematical objects, not just some class
of ‘constructive objects’.
This view, more or less, appears to have first been put forward by
Richman (1990, 1996). Taking the logic as the primary characteristic
of constructive mathematics, it does not reflect the primacy of
mathematics over logic that was part of the belief of Brouwer,
Heyting, Markov, Bishop, and other pioneers of constructivism. On
the other hand, it does capture the essence of constructive
mathematics in practice.
Thus one might distinguish between the ontological
constructivism of Brouwer and others who are led to constructive
mathematics through a belief that mathematical objects are mental
creations, and the epistemological constructivism of Richman
and those who see constructive mathematics as characterised by its
methodology, based on the use of intuitionistic logic. Of course, the
former approach to constructivism inevitably leads to the latter; and
the latter is certainly not inconsistent with a Brouwerian ontology.
From a technical point of view, there seem to be (at least) two ways
of handling the computational content and interpretation of
mathematics. One way uses classical logic. In order to avoid
decisions, such as whether or not a real number equals 0, that cannot
be made by a real computer, one then has to work within a carefully
specified algorithmic framework such as that of recursive function
theory. The second approach uses intuitionistic logic, which
automatically takes care of computationally inadmissible
decisions. It is then unnecessary to insist upon a closely
circumscribed algorithmic framework, so one can work in the style of
an analyst, algebraist, geometer, ... , the only constraints being
those imposed by intuitionistic logic.
References
- Beeson, Michael, 1985, Foundations of Constructive
Mathematics, Heidelberg: Springer-Verlag.
- Bishop, Errett, 1967, Foundations of Constructive
Analysis, New York: McGraw-Hill.
- Bishop, Errett, 1973, Schizophrenia in Contemporary
Mathematics, Amer. Math. Soc. Colloquium Lectures,
Missoula: University of Montana; reprinted in Errett Bisop:
Reflections on Him and His Research, Amer. Math. Soc. Memoirs
39.
- Bishop, E. and Bridges, D., 1985, , Grundlehren der
math. Wissenschaften 279, Heidelberg: Springer-Verlag.
- Bridges, D., Calder, A., Julian, W., Mines. R. and Richman, F.,
1982, "Picard's Theorem", Trans. Amer. Math. Soc.,
269(2), 513-520.
- Bridges, D. and Richman, F., 1987, Varieties of Constructive
Mathematics, London Math. Soc. Lecture Notes 97,
Cambridge: Cambridge University Press.
- Brouwer, L.E.J., 1907, Over de Grondslagen der Wiskunde,
Doctoral Thesis, University of Amsterdam, 1907; reprinted with
additional material, D. van Dalen (ed.), by Matematisch Centrum,
Amsterdam, 1981.
- Dummett, Michael, 1977, Elements of Intuitionism,
Oxford: Clarendon Press.
- Heyting, A., 1930, "Die formalen Regeln der
intuitionistischen Logik", Sitzungsber. preuss. Akad. Wiss. Berlin,
42-56.
- Hilbert, David, 1928, "Die Grundlagen der
Mathematik", Hamburger Mathematische Einzelschriften 5, Teubner,
Leipzig. Reprinted in English translation in van
Heijenoort 1967, in which the exact quotation appears on page
476.
- Kline, Morris, 1972. Mathematical Thought from Ancient to
Modern Times , vol 3, Oxford: Clarendon Press.
- Kushner, B., 1985, Lectures on Constructive Mathematical
Analysis, Providence RI: Amer. Math. Soc.
- Markov, A.A., 1954, Theory of Algorithms, Trudy
Mat. Istituta imeni V.A. Steklova, 42, Moskva: Izdatel'stvo
Akademii Nauk SSSR.
- Richman, Fred, 1983, "Church's Thesis Without Tears", J.
Symbolic Logic, 48, 797-803.
- -----, 1990, "Intuitionism as Generalization", Philosophia
Math, 5, 124-128.
- -----, 1996, "Interview with a Constructive Mathematician",
Modern Logic, 6, 247-271.
- Weihrauch, Klaus, 1996, "A Foundation for Computable Analysis", in
Combinatorics, Complexity, & Logic, D. Bridges, C. Calude, J.
Gibbons, S. Reeves, and I. Witten (eds.), Singapore: Springer-Verlag.
- -----, 2000, Computable Analysis, EATCS Texts in
Theoretical Computer Science, Heidelberg: Springer-Verlag.
Related Literature
- Bridges, Douglas, 1998, "Constructive Truth in Practice", in
Truth in Mathematics, H. Dales and G. Oliveri (eds.),
Oxford: Clarendon Press.
- Brouwer, L.E.J., 1908, "De onbetrouwbaarheid der logische principes",
Tijdschrift voor Wijsbegeerte, 2, 152-158.
- Goodman, N.D., and Myhill, J., "Choice Implies Excluded Middle",
Zeit. Logik und Grundlagen der Math, 24, 461.
- Hayashi, S., and Nakano, H., 1988, PX: A
Computational Logic, MIT Press, Cambridge MA.
- Heijenoort, Jean van, 1967, From Frege to
Gödel: A Source Book in Mathematical Logic 1879-1931,
Harvard University Press, Cambridge, Mass.
- Heyting, A., 1971, Intuitionism -- An Introduction, 3rd
edition, Amsterdam: North Holland.
- Hilbert, D., 1925, "Über das Unendliche", Mathematische
Annalen, 95, 161-190; translation, "On the Infinite", by
E. Putnam and G. Massey, in Philosophy of Mathematics: Selected
Readings, P. Benacerraf and H. Putnam (eds.), 134-151, Englewood
Cliffs, NJ: Prentice Hall, 1964.
- Mines, R., Richman, F., and Ruitenburg, W., 1988, A Course in
Constructive Algebra, Universitext, Heidelberg: Springer-Verlag.
- Myhill, John, 1973, "Some Properties of Intuitionistic
Zermelo-Fraenkel Set Theory", in Cambridge Summer School in
Mathematical Logic, A. Mathias and H. Rogers (eds.), Lecture
Notes in Mathematics, 337, Heidelberg: Springer-Verlag, 206-231.
- -----, 1975, "Constructive Set Theory", J. Symbolic
Logic, 40/3, 347-382.
- Martin-Löf, P., 1975, "An intuitionistic
theory of types: predicative part", in Logic Colloquium 1973
(H.E. Rose and J.C. Shepherdson, eds), 73-118, North-Holland,
Amsterdam.
- Richman, Fred, 2000, "The Fundamental Theorem of Algebra: A
Constructive Treatment Without Choice", Pacific J. Math.,
196, 213-230.
- Troelstra, A.S., 1978, "Aspects of Constructive Mathematics", in
Handbook of Mathematical Logic, J. Barwise (ed.), Amsterdam:
North-Holland.
- Troelstra, A.S., and van Dalen, D., 1988, Constructivity in
Mathematics: An Introduction (two volumes), Amsterdam: North
Holland.
- van Dalen, D., 1981, Brouwer's Cambridge Lectures on
Intuitionism, Cambridge: Cambridge University Press.
- -----, 1999, Mystic, Geometer and Intuitionist: The Life of
L.E.J. Brouwer, vol. I, Oxford: Clarendon Press.
- van Stigt, W.P., 1990, Brouwer's Intuitionism,
Amsterdam: North-Holland.
[Please contact the author with suggestions.]
Brouwer, Luitzen Egbertus Jan |
logic: intuitionistic
Copyright © 1997, 2002 by
Douglas Bridges
d.bridges@math.canterbury.ac.nz
A |
B |
C |
D |
E |
F |
G |
H |
I |
J |
K |
L |
M |
N |
O |
P |
Q |
R |
S |
T |
U |
V |
W |
X |
Y |
Z
 Table of Contents
Table of Contents
First published: November 18, 1997
Content last modified: March 26, 2002
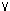 Q, where P and Q are syntactically
correct statements in some (formal or informal) language that a
mathematician can use? A natural -- although, as we shall see, not the
unique -- interpretation of this disjunction is that not only does (at
least) one of the statements P, Q hold, but also we
can decide which one holds. Thus just as mathematicians will assert
that P only when they have decided that P by
proving it, they may assert
P
Q, where P and Q are syntactically
correct statements in some (formal or informal) language that a
mathematician can use? A natural -- although, as we shall see, not the
unique -- interpretation of this disjunction is that not only does (at
least) one of the statements P, Q hold, but also we
can decide which one holds. Thus just as mathematicians will assert
that P only when they have decided that P by
proving it, they may assert
P 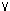 Q only when they either can decide -- that is, prove -- that
P or decide (prove) that Q.
Q only when they either can decide -- that is, prove -- that
P or decide (prove) that Q.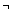 P,
of P. To decide that
P,
of P. To decide that
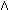
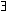 xP(x) means
xP(x) means
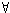 x
x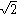
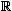 of real numbers:
of real numbers: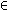
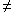 0),
0),
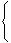
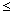 n
n
 a1a2a2
a1a2a2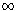
 (implies):
(implies):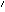 < b, computes an element x of S such
that x >
b
< b, computes an element x of S such
that x >
b > 0 there exists x such
that 0 < x < 1 and
|f(x)| <
> 0 there exists x such
that 0 < x < 1 and
|f(x)| <
 . Computing
. Computing
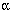 ,
,
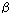 with
with
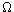 of ordered pairs
(
of ordered pairs
( )
of positive real numbers with the following two properties:
)
of positive real numbers with the following two properties:
 Table of Contents
Table of Contents